Normal distributions become more apparent (ie perfect) the finer the level of measurement and the larger the sample from a population You can also calculate coefficients which tell us about the size of the distribution tails in relation to the bump inExample 1 Sketch the gradient, and use that to draw the level curves, of the function ( ) We begin by finding the gradient 〈 〉 Since the function is explicit, we need only concern ourselves here with the derivatives for x and y At this point, we could choose a series of points that mark out a grid for our plane and begin to draw You can't find the tangent line of a function, what you want is the tangent line of a level curve of that function (at a particular point) $\endgroup$ – Hans Lundmark Sep 3 '18 at 549 Example regarding the LowenheimSkolem theorem (from Enderton's Logic)

Calculus Iii Functions Of Several Variables
Level curves examples
Level curves examples-Likewise, rv6= 0 Thus, the gradients are not zero and the level curves must be smooth Example 55 The gures below show level curves of uand vfor a number of functions In all cases, the level curves of uare in orange and those of vare in blue For each case we show the level curves separately and then overlayed on each otherOf level curves for the levels k n If the distance in the (x;y) plane between level curves for levels k n and k n 1 is large near a point P on the k n level curve, then the graph is not very steep there However, if the level curves are close together near P, then the graph is steeper near P Can you
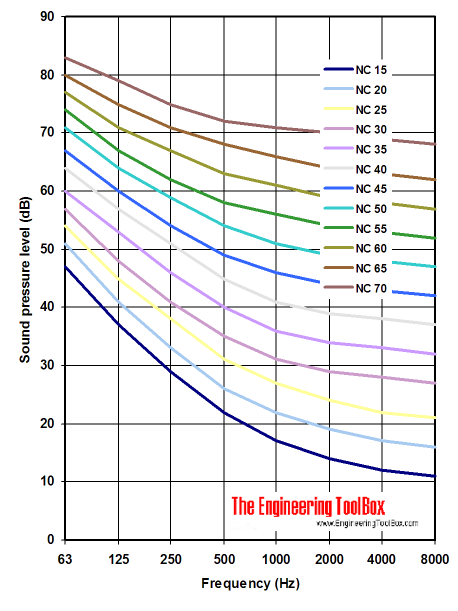



Nc The Noise Criterion
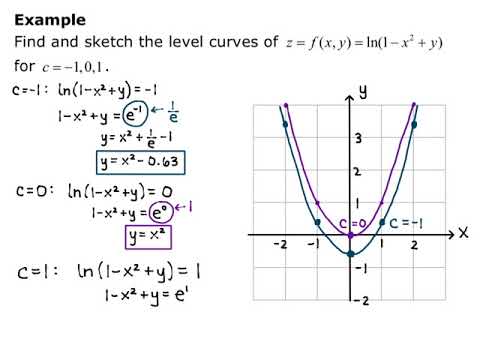
Level Curves and Surfaces Example 2 In mathematics, a level set of a function f is a set of points whose images under f form a level surface, ie a surface such that every tangent plane to the surface at a point of the set is parallel to the level set Its graph is shown below From the side view, it appears that the minimum value of this function is around 500 Example 4 f (x,y)=2e (x1)2y2 3e (x2)2 (y1)2 2e (x1)2 (y2)2 A level curve of a function f (x,y) is a set of points (x,y) in the plane such that f (x,y)=c for a fixed value c Example 5We have done this in Example 4 at level curves Unfortunately, sometimes it is not possible to rewrite the level curve Unfortunately, sometimes it is not possible to rewrite the level curve However, even in that case we can determine the slope of the tangent line to the level curve by the use of the following rule
Perpendicular to the Level Curve TheoremThe gradient isalways perpendicular to the level curve through its tail Proof We will only show this for a surfa ce z f(x,y) whose level curve c f(x,y) can be parameterized by(x(t),y(t)) Then atangent vector on the level curve can be described by (x'(t),y'(t)) ff Next, the gradient is f(x,y) , xyThe AD curve relationship between the price level and real GDP demanded, holding everything else constant A change in the price level not caused by a component of real GDP changing results in a movement along the AD curve A change in some component of aggregate demand, on the other hand, will shift the AD curveThis Service Has Been Retired Faculty profile information has been migrated to UMassD Sites and the University's Directory
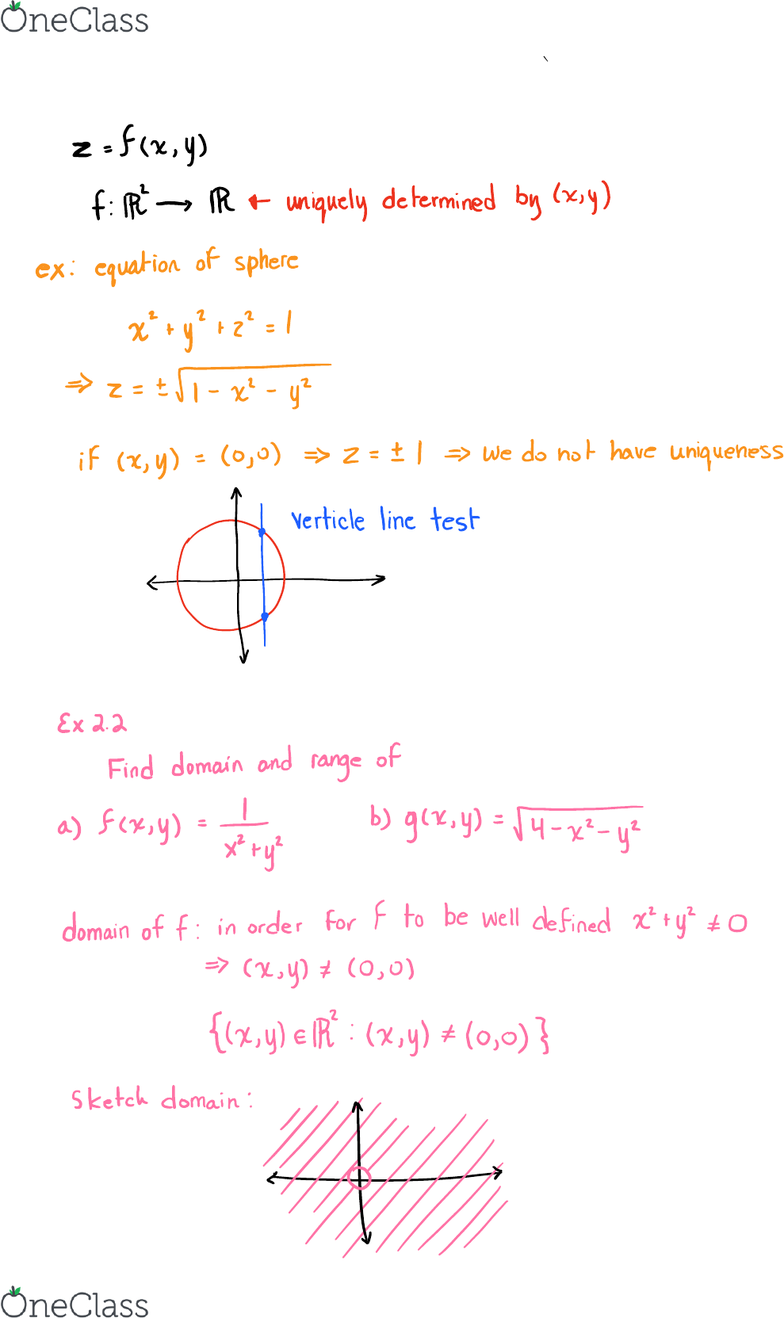
In your first example, the proper solution is $$y=\pm \sqrt{kx^2}$$ You left out the plusorminus That is not a small thing there are usually two values of $y$ for each $x$, and that greatly affects the plotting of the curvesU(x;y) = cas the curve which represents the easiest walking path, that is, altitude does not change along that route The altitude is conserved along the route, hence the terminology conservation law Other examples of level curves are isobars and isotherms An isobar is a planar curve where the atmospheric pressure is constant An isotherm is a planar curve along which the temperature isLevel Curves We have a multivariable function (more than one variable) that is a square root function A level curve is a twodimensional curve got by equating a constant value to the function
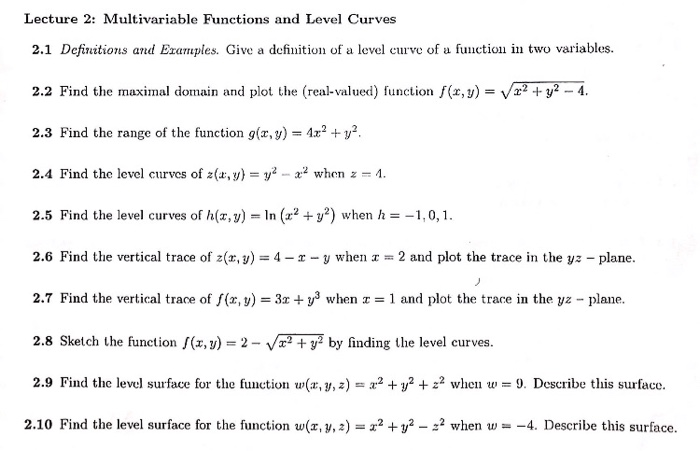



Solved Multivariable Functions And Level Curvesi M Having A Chegg Com




Plotting Using Contour In Python Epythonguru
For example, when c 1= 0, the level curve is which is a circle of radius 8 27 Figure 1310 shows the nine level curves for the hemisphere cont'd Figure 1310 Example 3 – Solution 28 One example of a function of two variables used in economics is the CobbDouglas production functionWhen we talk about the graph of a function with two variables defined on a subset D of the xyplane, we mean zfxy xy D= (, ) ,( )∈ If c is a value in the range of f then we can sketch the curve f(x,y) = cThis is called a level curve A collection of level curves can give a good representation of the 3d graphA level curve in R2 is a set of points C= f(x;y) 2R2 jf(x;y) = cg Example 11 The unit circle x2 y2 = 1 can be described as a level curve by being the zero set of f(x;y) = x2 y2 1 Level curves in higher dimension such as R3 require more de ning equations Example 12 The xaxis in R3 is described as a level curve by C= f(x;y;z) 2R3 jy= 0



Ucm Gravity
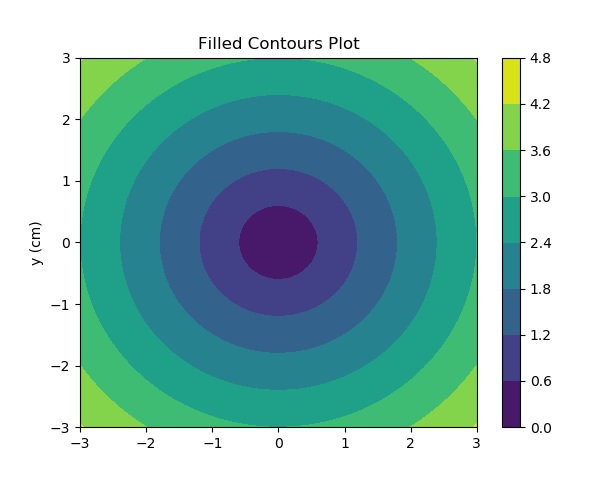



Matplotlib Contour Plot
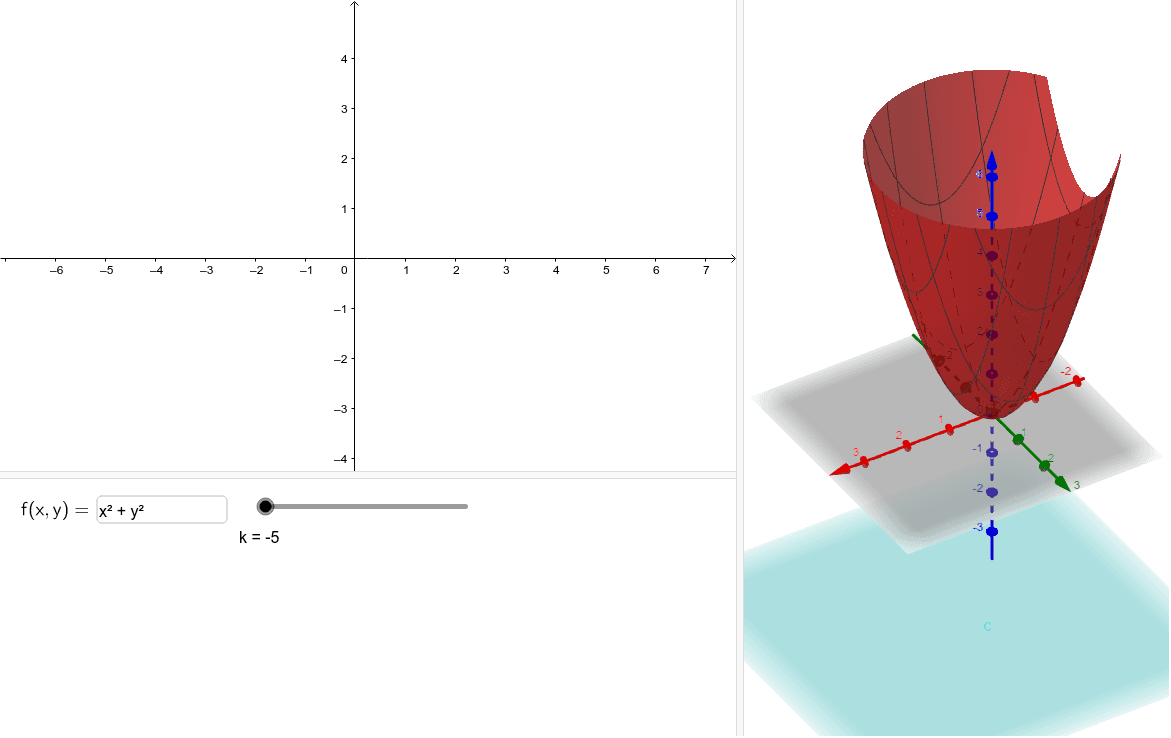
It looks much like a topographic map of the surfaceA level curve of a function is curve of points where function have constant values,level curve is simply a cross section of graph of function when equated to some constant values ,example a function of two variables say x and y ,then level curve is the curve of points (x,y) ,where function have constant value Can be better understood by an example Find the level curves of heights CIn the simplest case, = 2 2, an intersection of this surface with a plane =𝑘 forms a level curve that is a circle Thus, the contour map of this paraboloid will be concentric circles centered at the origin The paraboloid = 2 2 intersected by this surface with a plane =𝑘 forms a circle



Math 15 Lecture 7 Level Curves And Contour Plots Oneclass



Section 13 1 Level Curves Youtube
The level curves f(x,y) = k are just the traces of the graph of f in the horizontal plane z=k projected down to the xyplane Figure 1 Relation between level curves and a surface k is variating acording to 5015 One common example of level curves occurs in topographic maps of mountainous regions, such as the map in Figure 2 The level curves are curves of constant elevation of theLevel curves and contour plots are another way of visualizing functions of two variables If you have seen a topographic map then you have seen a contour plot Example To illustrate this we first draw the graph of z = x2 y2 On this graph we draw contours, which are curves at a fixed height z = constant For example the curve at height z = 1 is the circle x2 y2 = 1 On the graph we haveFor example, "tallest building" Search for wildcards or unknown words Put a * in your word or phrase where you want to leave a placeholder For example, "largest * in the world" Search within a range of numbers Put between two numbers For example, camera $50$100 Combine searches Put "OR" between each search query For example, marathon



Problem On Gradient Directional Derivative And Level Curves Leading Lesson



Level Curves Geogebra
R251pdf Curves in R2 Graphs vs Level Sets Graphs(y = f(x The graph of f R \u2192 R is(x y \u28 R2 y = f(x Example When we say \u1cthe curve y = x2,\u1dFigure 10 Contour Plot with Highlighted Level Curve 52 Example 2 Contour Plot for z = x 2 9 y 4 This example is a contour plot for a hyperboloid Details for each step are omitted, since they were discussed for example 1 The steps to create the contour plot are Step 1 Define the function z= f(x;y) and solve it for y in terms of x and zExample, the set Sis in R2 This gure also illustrates the fact that a ball in R2 is just a disk and its boundary18 23 An example of in nitely many alternative optimal solutions in a linear programming problem The level curves for z(x 1;x 2) = 18x 1 6x 2 are parallel to one face of the polygon boundary of the feasible region Moreover



Functions Of Several Variables Ximera




Graphs And Level Curves


